how to find the degree of a polynomial

Degree of Polynomial

Polynomials are one of the significant concepts of mathematics, and so is the caste of polynomials, which determines the maximum number of solutions a function could take and the number of times a role volition cantankerous the 10-axis when graphed. Information technology is the highest exponential power in the polynomial equation. Let us larn in detail near this concept and how to find the degree of a polynomial.

1.	What is Caste of a Polynomial?
two.	How to Observe the Degree of a Polynomial?
three.	Polynomials Based on Degree
4.	Degree of a Polynomial Applications
5.	FAQs on Degree of a Polynomial

What is Degree of Polynomial?

The degree of a polynomial is the highest exponential power in the polynomial equation. Merely variables are considered to bank check for the degree of any polynomial, coefficients are to be ignored. For an n^th degree polynomial function with real coefficients and x as the variable having the highest ability n, where n takes whole number values, the degree of a polynomial in standard grade is given every bit p (x) = a_ntenⁿ + a_n-1ten^n-1 + a_n-2x^n-2 + ... + a₁x¹ + a₀.

Degree of a Polynomial Definition:

The caste of a polynomial is the greatest power of a variable in the polynomial equation. To make up one's mind the caste of a polynomial function, simply the terms with variables are considered to observe out the degree of polynomial. The highest exponential power of the variable term in the polynomial indicates the degree of that polynomial.

Look at the polynomial function given below, where the highest power of x is n. Hence, n is the degree of polynomial in this function.

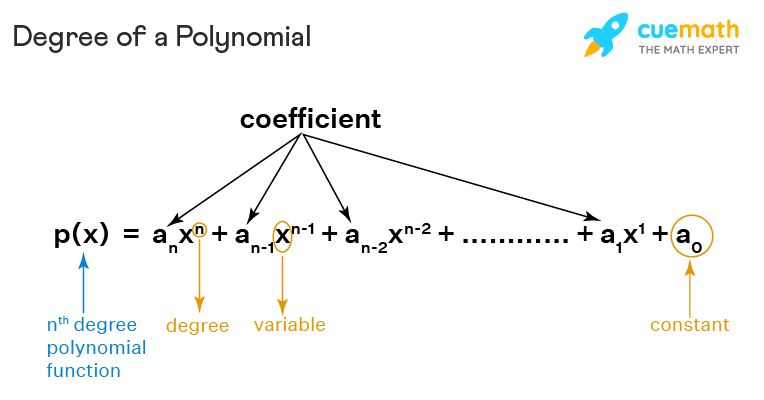
degree of polynomial

How to Find the Caste of Polynomial?

Consider the polynomial: p(ten): 2x⁵−12x³+3x−π. The term with the highest power of x is 2x⁵ and the corresponding (highest) exponent is five. Therefore, we volition say that the degree of this polynomial is v. Thus, the degree of a polynomial is the highest power of the variable in the polynomial. We tin correspond the degree of a polynomial by Deg(p(x)). Given below are some examples:

Deg(xⁱⁱⁱ+1) = 3
Deg(i+x+x^two+x^three+...+x^l) = 50
Deg(10+π³) = 1

Note that the degree is the highest exponent of the variable term, so even though the exponent of π is 3 (refer to the last example given higher up), that is irrelevant to make up one's mind the degree of the polynomial.

Degree of a Zero Polynomial

When all the coefficients are equal to goose egg, the polynomial is considered to exist a nothing polynomial. And so, the caste of the zero polynomial is either undefined or defined in a way that is negative (-1 or ∞).

Degree of a Abiding Polynomial

A constant polynomial (P(10) = c) has no variables. Since there is no variable, there is no power to it. Thus, the degree of the constant polynomial is goose egg. For example: For half dozen or 6x⁰, degree = 0.

Degree of a Polynomial With More 1 Variable

The degree of a polynomial with more than than one variable tin exist calculated by adding the exponents of each variable in it. For example: 5x³ + 6xⁱⁱy² + 2xy.

5xⁱⁱⁱ has a degree of three (x has an exponent of 3).
6x²y^two has a caste of iv (x has an exponent of 2, y has 2, so two+2=four).
2xy has a degree of 2 (x has an exponent of 1, y has 1, so ane+1=two).

The largest caste out of those is four, so the polynomial has a degree of 4.

Polynomials Based on Caste

Each of the polynomials has a specific caste and based on that they accept been assigned a specific name. Let's allocate the polynomials based on the degree of polynomial with examples.

Polynomials	Caste	Examples
Abiding Polynomial	Polynomials with Degree 0	iii
Linear Polynomial	Polynomials with Degree one	x + eight
Quadratic Polynomial	Polynomials with Degree 2	3x^two - 4x + seven
Cubic Polynomial	Polynomials with Degree iii	2x³ + 3x² + 4x + six
Quartic Polynomial	Polynomials with Degree iv	x^iv-xvi
Quintic Polynomial	Polynomials with Degree five	4x^five+ 2x³ - xx

Caste of a Polynomial Applications

Given below are a few applications of the degree of a polynomial:

To determine the maximum number of solutions that a office could have.
To determine the maximum number of times a function will cantankerous the ten-axis when graphed.
To check whether the polynomial expression is homogeneous, determine the caste of each term. When the degrees of the term are equal, so the polynomial expression is homogeneous and when the degrees are not equal, then the expression is said to be non-homogenous. For example, in 4x³ + 3xy²+8y³, the degree of all the terms is three. Hence, the given example is a homogeneous polynomial of degree 3.

Degree of Polynomial Tips and Tricks:

In social club to find the degree of a polynomial, you lot tin follow these steps:

Identify each term of the given polynomial.
Combine all the like terms, the variable terms; ignore constant terms.
Accommodate those terms in descending gild of their powers.
Find the term with the highest exponent and that defines the degree of the polynomial.

Important Notes:

Degree of a polynomial with but one variable: The largest exponent of the variable in the polynomial (using Descartes' rule of signs)
Degree of a polynomial with more than than 1 variable: Add together the exponents of each variable given in a term, and find which term has the greatest caste. That will exist considered every bit the degree of the polynomial.
Degree of a rational expression: Accept the caste of the top (numerator) and decrease the degree of the bottom (denominator).
The degree of any polynomial expression with a square root such as 3√10 is 1/2.

Related Articles

Cheque these interesting articles related to the concept of the degree of polynomials in math.

nth Caste Polynomial
Types of Polynomials
Polynomials in I Variable
Polynomial Degree Calculator

Caste of Polynomial Examples

Example 1: Decide the leading coefficient and the degree of the polynomial of the post-obit expression 5x² - 20x - 20.

Solution:

Given a polynomial expression, 5xⁱⁱ - 20x - 20. The highest exponent of the variable x is 2, and so the caste of the expression is 2. The coefficient with the highest exponent will exist the leading coefficient of the expression, and so the leading coefficient is v.

Therefore, degree = two and leading coefficient = 5.
Case 2: Find the degree of the polynomial 5x⁴ + 3x² - 7x⁵ + x^vii.

Solution:

In lodge to find the degree, check each term of the given polynomial. All are unlike terms with ten as a variable. Adjust these terms in descending order of their powers, which gives 10^vii - 7x^v + 5x^four+ 3x². The term with the greatest or highest exponent is x⁷. Therefore, the caste of the polynomial is vii.
Case iii: Find a fourth-caste polynomial satisfying the following conditions: has roots (x-two), (x+5) and should exist divisible by 4xⁱⁱ.

Solution:

Nosotros are already familiar with the fact that a 4th-degree polynomial is a polynomial with degree 4. Also, we know that nosotros can detect a polynomial expression by its roots.
Commencement status: (x-2) (ten+5) = ten(x+five) - 2(ten+5) = x²+5x-2x-x = x^two+3x-10.
Second condition: (x²+3x-10)(4x²) = x².4x² + 3x.4xⁱⁱ - 10.4x²= 4x⁴+12x³-40x²

Therefore, the required polynomial is 4x^four+ 12x³- 40x².

go to slidego to slidego to slide

Breakup tough concepts through unproblematic visuals.

Math will no longer be a tough field of study, especially when you sympathise the concepts through visualizations.

Volume a Free Trial Grade

Practice Questions on Degree of a Polynomial

go to slidego to slide

FAQs on Degree of a Polynomial

What is the Degree of a Polynomial?

The degree of a polynomial is the highest caste of the variable term with a non-nil coefficient in the polynomial.

What is the Degree of a Quadratic Polynomial?

Quadratic polynomials are characterized as polynomials with degree ii. Thus, the degree of a quadratic polynomial is 2.

What is Caste 3 Polynomial?

Degree 3 polynomials are known as cubic polynomials. They have 3 as the highest exponent of the variable. 1 example of a degree of the polynomial three is x³ + 5x - 20.

What is the Degree of the Zip Polynomial?

A zip polynomial has all its variable coefficients equal to zero. Information technology is a constant polynomial having a value of 0. Thus, the degree of the null polynomial is undefined.

What is the Caste of Polynomial v√10?

For the polynomial five√x, the exponent with variable x is 1/two. Thus, the degree of polynomial v√x is 1/ii.

What is the Degree of the Polynomial five√3?

The degree of polynomial 5√3 is nothing as at that place is no variable and the degree of any polynomial is defined by the highest exponential power of its variable term.

Why is the Caste of a Polynomial Important?

The caste of a polynomial function has peachy importance as it determines the maximum number of solutions that a role could have and the maximum number of times a function crosses the ten-axis on graphing it.

Source: https://www.cuemath.com/algebra/degree-of-a-polynomial/

Posted by: gillespieextesed.blogspot.com