how to find equation of parabola with focus and directrix

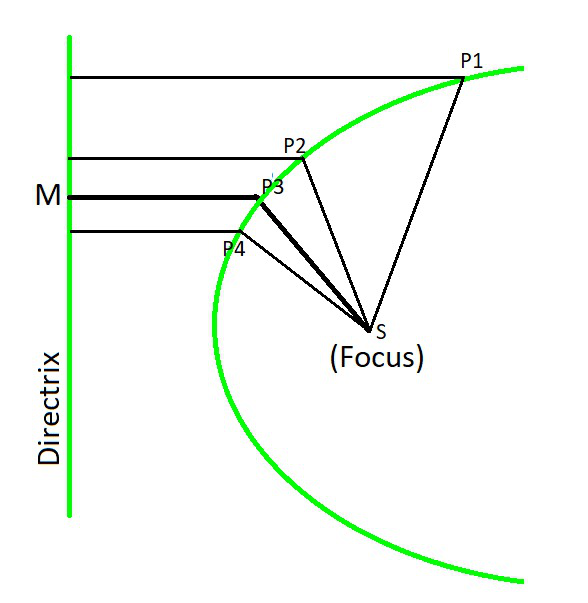
In mathematics, a parabola is the locus of a bespeak that moves in a airplane where its distance from a fixed point known as the focus is always equal to the altitude from a fixed straight line known as directrix in the aforementioned airplane. Or in other words, a parabola is a plane curve that is most in U shape where every point is equidistance from a fixed signal known every bit focus and the straight line known as directrix. Parabola has just 1 focus and the focus never lies on the directrix. As shown in the below diagram, where P_aneM = P₁S, P_iiK = P₂Due south, P_iiiM = P₃S, and P_fourM = P₄S.

Equation of the parabola from focus & directrix

Now we will learn how to find the equation of the parabola from focus & directrix. So, let South be the focus, and the line ZZ' be the directrix. Draw SK perpendicular from S on the directrix and bifurcate SK at V. Then,

VS = VK

The distance of V from the focus = Distance of V from the directrix

5 lies on the parabola, So, SK = 2a.

And then, VS = VK = a

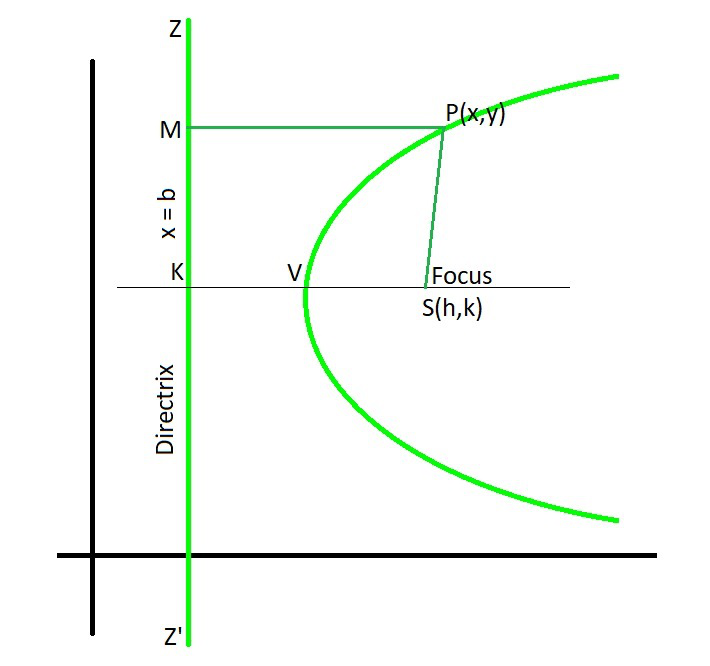
Permit'southward accept V equally vertex, VK is a line perpendicular to ZZ' and parallel to the x-centrality. Then, the coordinates of focus Due south are (h, thou) and the equation of the directrix ZZ' is x = b. PM is perpendicular to directrix x = b and bespeak G volition be (b, y)

Let us considered a bespeak P(x, y) on the parabola. Now, join SP and PM.

Equally we know that P lies on the parabola

So, SP = PM (Parabola definition)

SPⁱⁱ = PM²

(x – h)ⁱⁱ + (y – 1000)^two = (x – b)² + (y – y)^two

10² – 2hx + h² + (y-chiliad)ⁱⁱ = x² – 2bx + b²

Add together (2hx – b²) both side, we get

x² – 2hx + hⁱⁱ + 2hx – b² + (y-k)² = x² – 2bx + b^two + 2hx – b²

2(h – b)x = (y-k)² + h² – b^two

Divide equation past 2(h – b), we get

x = $\frac{(y-k)^2}{2(h-b)} + \frac{(h-b)(h+b)}{2(h-b)}$

x = $\mathbf{\frac{(y-k)^2}{2(h-b)} + \frac{(h+b)}{2}}$ ………………..(ane)

Similarly when directrix y = b, we get

y = $\mathbf{\frac{(x-h)^2}{2(k-b)} + \frac{(k+b)}{2}}$ ………………..(2)

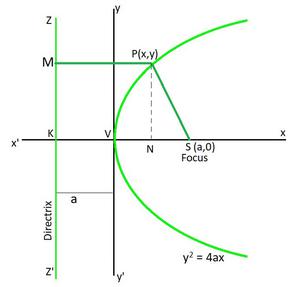
When V is origin, VS as ten-axis of length a. And so, the coordinates of S will exist (a, 0), and directrix ZZ' is x = -a.

h = a, k = 0 and b = -a

Using the equation (one), we get

10 = $\frac{(y-0)^2}{2(a-(-a))} + \frac{(a+(-a))}{2}$

x = $\frac{y^2}{4a}$

y^two = 4ax

It is the standard equation of the parabola.

Note: The parabola has two real foci situated on its axis one of which is the focus South and the other lies at infinity. The corresponding directrix is also at infinity.

Tracing of the parabola yⁱⁱ = 4ax, a>0

The given equation tin be written as y = ± 2 $\sqrt{ax}$ , we detect the following points from the equation:

Symmetry: The given equation states that for every positive value of x, there are two equal and reverse value of y.
Region: The given equation states that for every negative value of x, the value of y is imaginary which means no part of the curve lies to left of the y-axis.
Origin: Origin is the bespeak from where the bend passes through and the tangent at the origin is ten = 0 i.eastward., y-axis.
Portion occupied: As x⇢∞, y⇢∞. Hence the curve extends to infinity to the right of the axis of y.

Some other standard forms of the parabola with focus and directrix

The simplest form of the parabola equation is when the vertex is at the origin and the axis of symmetry is along with the x-axis or y-axis. Such types of parabola are:

i. y² = 4ax

Here,

Coordinates of vertex: (0, 0)
Coordinates of focus: (a, 0)
Equation of the directrix: x = -a
Equation of axis: y = 0
Length of the latus rectum: 4a
Focal distance of a point P(x, y): a + x

two. x² = 4ay

Here,

Coordinates of vertex: (0, 0)
Coordinates of focus: (-a, 0)
Equation of the directrix: x = a
Equation of axis: y = 0
Length of the latus rectum: 4a
Focal distance of a point P(x, y): a – x

3. y² = – 4ay

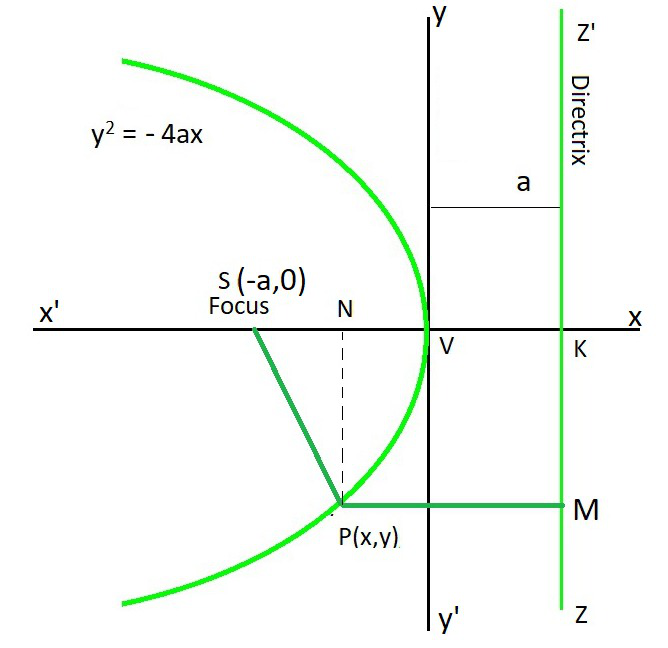
Here,

Coordinates of vertex: (0, 0)
Coordinates of focus: (0, a)
Equation of the directrix: y = -a
Equation of centrality: x = 0
Length of the latus rectum: 4a
Focal altitude of a point P(x, y): a + y

4. 10² = – 4ay

Hither,

Coordinates of vertex: (0, 0)
Coordinates of focus: (0, -a)
Equation of the directrix: y = a
Equation of axis: ten = 0
Length of the latus rectum: 4a
Focal distance of a bespeak P(x, y): a – y

Sample Problems

Question one. Find the equation of the parabola whose focus is (-4, ii) and the directrix is x + y = 3.

Solution:

Let P (x, y) be any indicate on the parabola whose focus is (-four, 2) and the directrix x + y – 3 = 0.

As we already know that the distance of a betoken P from focus = altitude of a signal P from directrix

And then, √(x + 4)² + (y – 2)²= $|\frac{x+y-3}{\sqrt{1^2+1^2}}|$

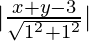
On squaring both side we get

(x + 4)² + (y – 2)²= $|\frac{x+y-3}{\sqrt{2}}|^2$

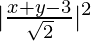
ii((10ⁱⁱ + 16 + 8x) + (y²+ 4 – 4y)) = ten² + y² + 9 +2xy – 6x – 6y

2(x² + 20 + 8x + y²– 4y) = x² + y² + 9 +2xy – 6x – 6y

2x² + 40 + 16x + 2y²– 8y = 10² + y² + ix +2xy – 6x – 6y

x^two + yⁱⁱ + 2xy + 10x – 2y + 31 = 0

Question two. Detect the equation of the parabola whose focus is (-4, 0) and the directrix x + half-dozen = 0.

Solution:

Permit P (x, y) exist whatsoever point on the parabola whose focus is (-4, 0) and the directrix ten + six = 0.

As we already know that the distance of a point P from focus = distance of a point P from directrix

Then, √(x + 4)² + (y )^two= $|\frac{x+6}{\sqrt{1^2+1^2}}|$

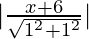
On squaring both side we get

(x + 4)² + (y)²= $|\frac{x+6}{\sqrt{2}}|^2$

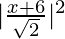
2x^two + 32 + 16x + 2yⁱⁱ= ten^two + 36 + 12x

10^two + 2y² – 4 + 14x = 0

Question 3. Find the equation of the parabola with focus (4, 0) and directrix x = – 3.

Solution:

Since the focus (iv, 0) lies on the x-axis, the 10-centrality itself is the centrality of the parabola.

Hence, the equation of the parabola is of the grade either

y^two= 4ax or y²= – 4ax.

Since the directrix is 10 = – 3 and the focus is (4, 0),

the parabola is to be of the form y^two= 4ax with a = 4.

Hence, the required equation is

yⁱⁱ = 4(4)ten

yⁱⁱ = 16x

Question four. Detect the equation of the parabola with vertex at (0, 0) and focus at (0, 4).

Solution:

Since the vertex is at (0, 0) and the focus is at (0, 5) which lies on y-axis, the y-axis is the axis of the parabola.

Hence, the equation of the parabola is tenⁱⁱ= 4ay.

Hence, we have x^two = four(iv)y, i.e.,

x² = 16y

Focus & directrix of a parabola from the equation

Now we will learn how to find the focus & directrix of a parabola from the equation.

So, when the equation of a parabola is

y – k = a(x – h)²

Hither, the value of a = 1/4C

Then the focus is (h, chiliad + C), the vertex is (h, k) and the directrix is y = g – C.

Sample Examples

Question 1. y^two = 8x

Solution:

The given parabola is of the form yⁱⁱ = 4ax, where

4a = 8

a = 2

The coordinates of the focus are (a,0), i.e. (two,0)

and, the equation of the directrix is

x = -a, i.e. x = -2

Question 2. yⁱⁱ – 8y – x + 19 = 0

Solution:

Past rearranging, we go

y² – 8y + xvi – x + 3 = 0

y² – 8y + 16 = 10 – iii

x = (y-4)^two + 3

Comparing with eq(one), we conclude

k = 4

2(h-b) = i ……………(I)

$\frac{(h+b)}{2}$ = 3 ……………(II)

Solving (I) and (II), we get

h = $\frac{13}{4}$ and b = $\frac{11}{4}$

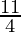
Hence, Focus is (h,chiliad) = ( $\frac{13}{4}$ ,iv)

and, directrix x = b = $\frac{11}{4}$

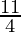
Question iii. Find focus, directrix and vertex of the post-obit equation: y = x² – 2x + iii

Solution:

Past rearranging, we go

y =x^two – 2x + four – 1

y =(x-1)² + 2

Comparing with eq(iv), we conclude

h = ane

y₁ = ii

two(k-b) = 1 ……………(I)

$\frac{(k+b)}{2}$ = 2 ……………(II)

Solving (I) and (2), we go

k = $\frac{9}{4}$

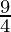
and b = $\frac{7}{4}$

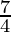
Hence, Focus is (h,k) = ( ane, $\frac{9}{4}$ ),

directrix y = b = $\frac{7}{4}$

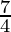
and, vertex (h, y_i) = (i,2)